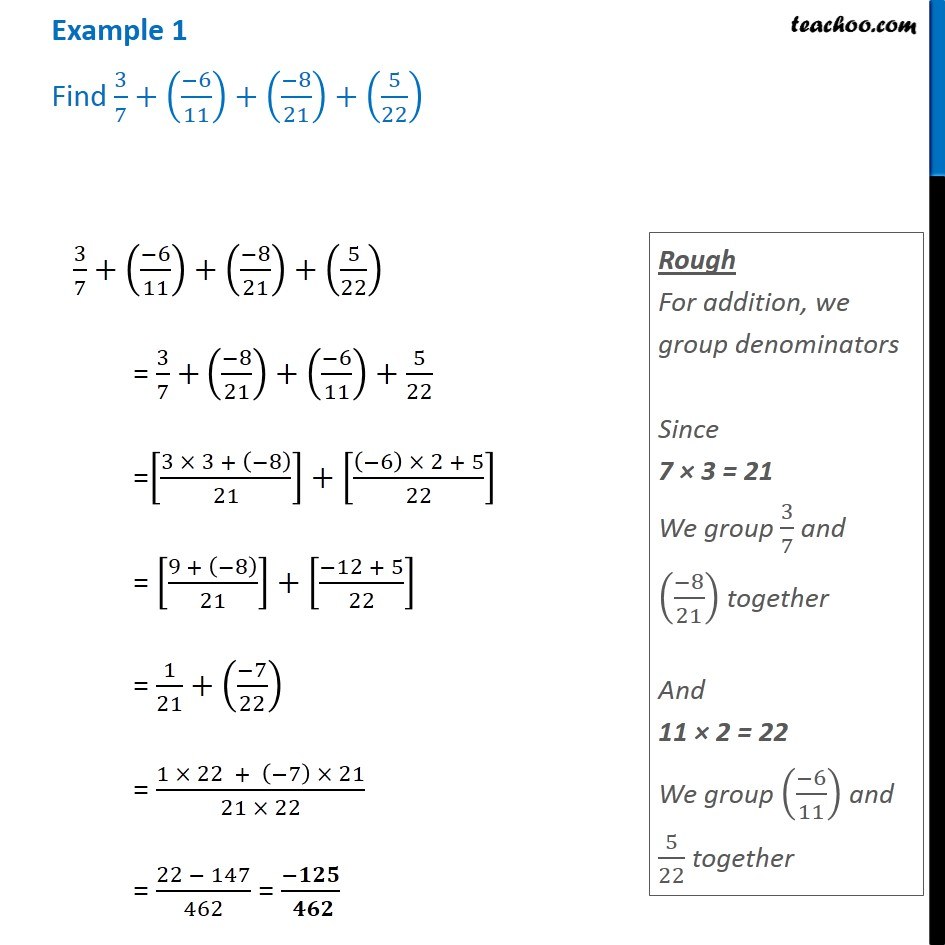
√ １−６ 158355

24 Use a General Strategy to Solve Linear Equations;

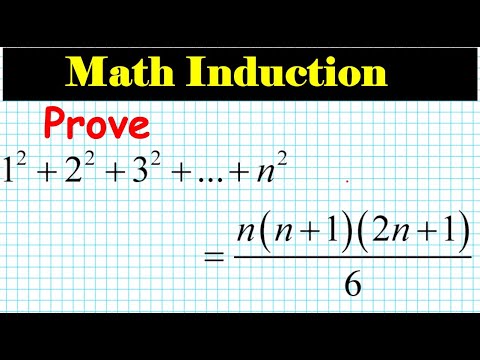
１−６-Created by T Madas Created by T Madas Question 19 (***) f x x x( ) ≡ 6 72, x∈ The remainder when f x( ) is divided by (x a−) is the same as that when f x( ) is divided by (x a2), where a is a non zero constant Find the value of a C2N , 1 6 a = Question (***) A cubic function is defined in terms of the positive constant k asDivide the numerator by −1 Remember to change the sign of all terms when dividing by −1 Practice 1 Simplify a 45 b 125 c 48 d 175 e 300 f 28 g 72 h 162 2 Simplify a 72 162 b 45 2 5− Check you have c 50 8− d 75 48− e 2 28 28 f 2 12 12 27− 3 Ex p and and simplify a ( 2 3)( 2 3) − b (3 3)(5 12)− − c (4 5)( 45 2) d (5 2)(6 8)−

１−６のギャラリー

各画像をクリックすると、ダウンロードまたは拡大表示できます

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

ページ番号をクリックして他の画像を表示し、画像をクリックして画像のダウンロードリンクを取得します

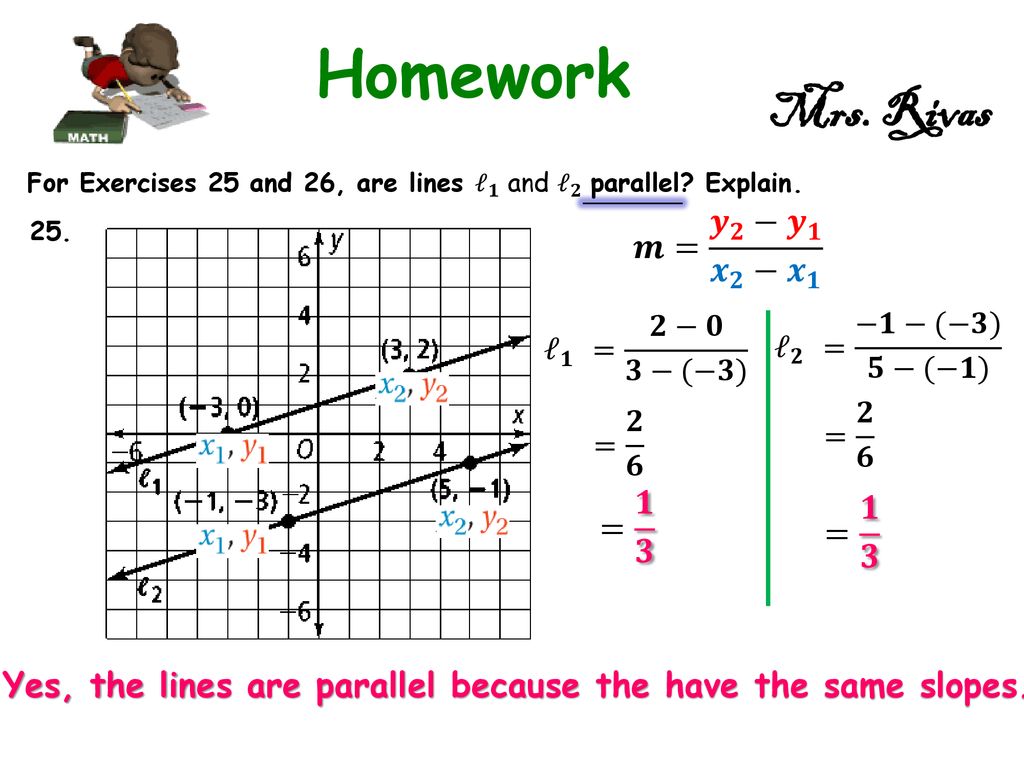
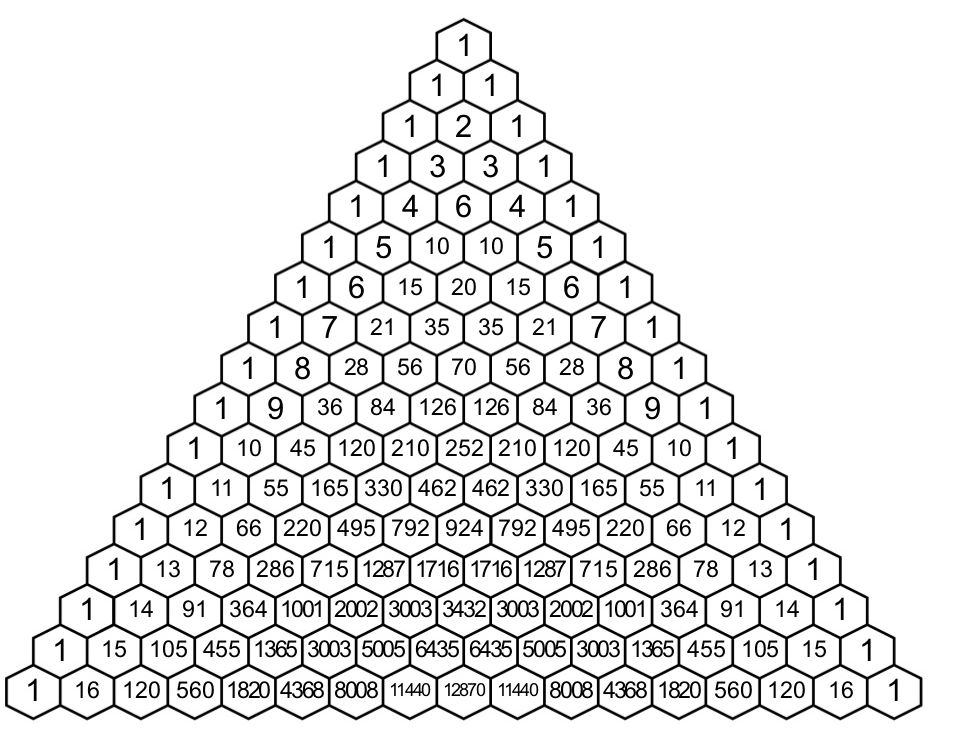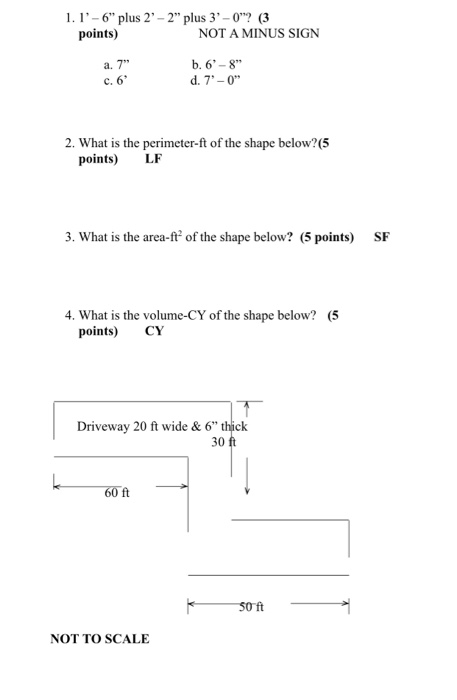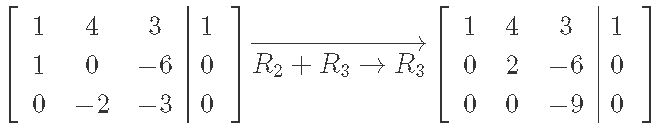「１−６」の画像ギャラリー、詳細は各画像をクリックしてください。

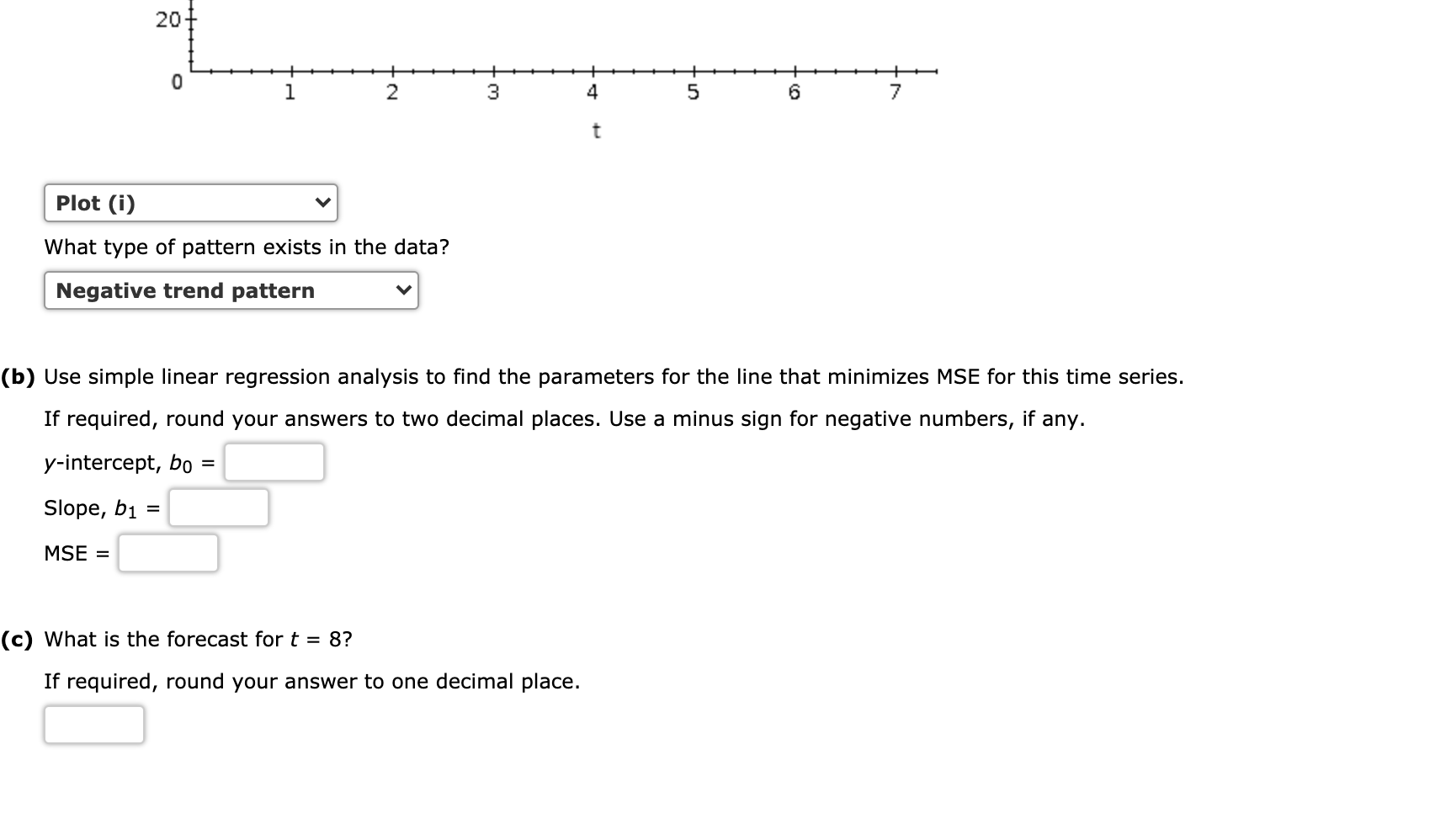−1 1 ,~v 2 = 1 0 1 ,~v 3 = 1 1 2 To apply the GramSchmidt, we first need to check that the set of vectors are linearly independent 1 1 1 −1 0 1 1 1 2 = 1(0−1)−1((−1)(2)−(1)(1))1((−1)(1)−0) = 1 6= 0 Therefore the vectors are linearly independent GramSchmidt algorithm Step 1 Let ~u 1 = ~v 1 = 1 −1 1 ~e 1 = ~u 1 ~u 11 x 2 (1)(−1)(−3) 3!